Prezentacje/Presentations 91
pól, nazywanych komórkami. Każda komórka może zna-
leźć się w jednym z kilku stanów, których może być do
wolnie dużo, jednak ich liczba musi być skończona. Zbu
dowany w ten sposób automat komórkowy podda wa ny
jest cyklicznym przekształceniom, podczas których zmie
niają się stany poszczególnych komórek. Stan ko mórki po
przekształceniu zależy wyłącznie od stanu, w ja kim znaj-
dowały się otaczające ją komórki (tzw. są siedztwo) przed
przekształceniem. Podczas przekształcenia stany wszyst-
kich komórek zmieniają się jednocześnie zgodnie z za
sadami przyjętymi dla konkretnego automatu komór
kowego [1].
W 1970 r. brytyjski matematyk John Conway opraco-
wał automat komórkowy o nazwie Gra w życie (ang. Game
of life). Składa się on ze zbioru prostych zasad, które po
zwalają na generowanie skomplikowanych struktur oraz
ich ewolucję w kolejnych cyklach przekształceń. Plansza
Gry to nieskończona płaszczyzna ortogonalnej siatki,
a wszystkie znajdujące się na niej komórki muszą być
w jednym z dwóch dozwolonych stanów – komórka żywa
bądź martwa. Pierwotny stan komórek określa gracz po
przez założenie, które z nich są żywe. Wygenerowa na
w ten sposób struktura jest następnie przekształcana w ko
lejnych cyklach, a stan komórki w następnej generacji
jest wynikową stanu jej sąsiedztwa w generacji po
przedniej. Na sąsiedztwo każdej komórki składa się osiem
przylegających do niej komórek – po cztery prostopadle
oraz po przekątnej [2]. Przekształcenia odbywają się na
podstawie następujących zasad:
– Narodziny. Komórka, która jest martwa w generacji
x, staje się żywa w generacji x+1, jeżeli dokładnie trzy
komórki z jej sąsiedztwa w generacji x były żywe.
– Śmierć z powodu zatłoczenia. Komórka, która jest
żywa w generacji x i ma jednego lub mniej sąsiadów w tej
generacji, staje się martwa w generacji x+1.
– Śmierć z powodu samotności. Komórka, która jest
żywa w generacji x i ma czterech lub więcej sąsiadów
w tej generacji, staje się martwa w generacji x+1.
– Przetrwanie. Komórka, która jest żywa w generacji x,
przetrwa żywa do generacji x+1 tylko wtedy, gdy w gene-
racji x będzie miała dwóch lub trzech sąsiadów [3].
Oprócz regulowania przemian w chaotycznych struk-
turach zasady Gry w życie generują wiele konfiguracji
charakteryzujących się złożonym zachowaniem. Są wśród
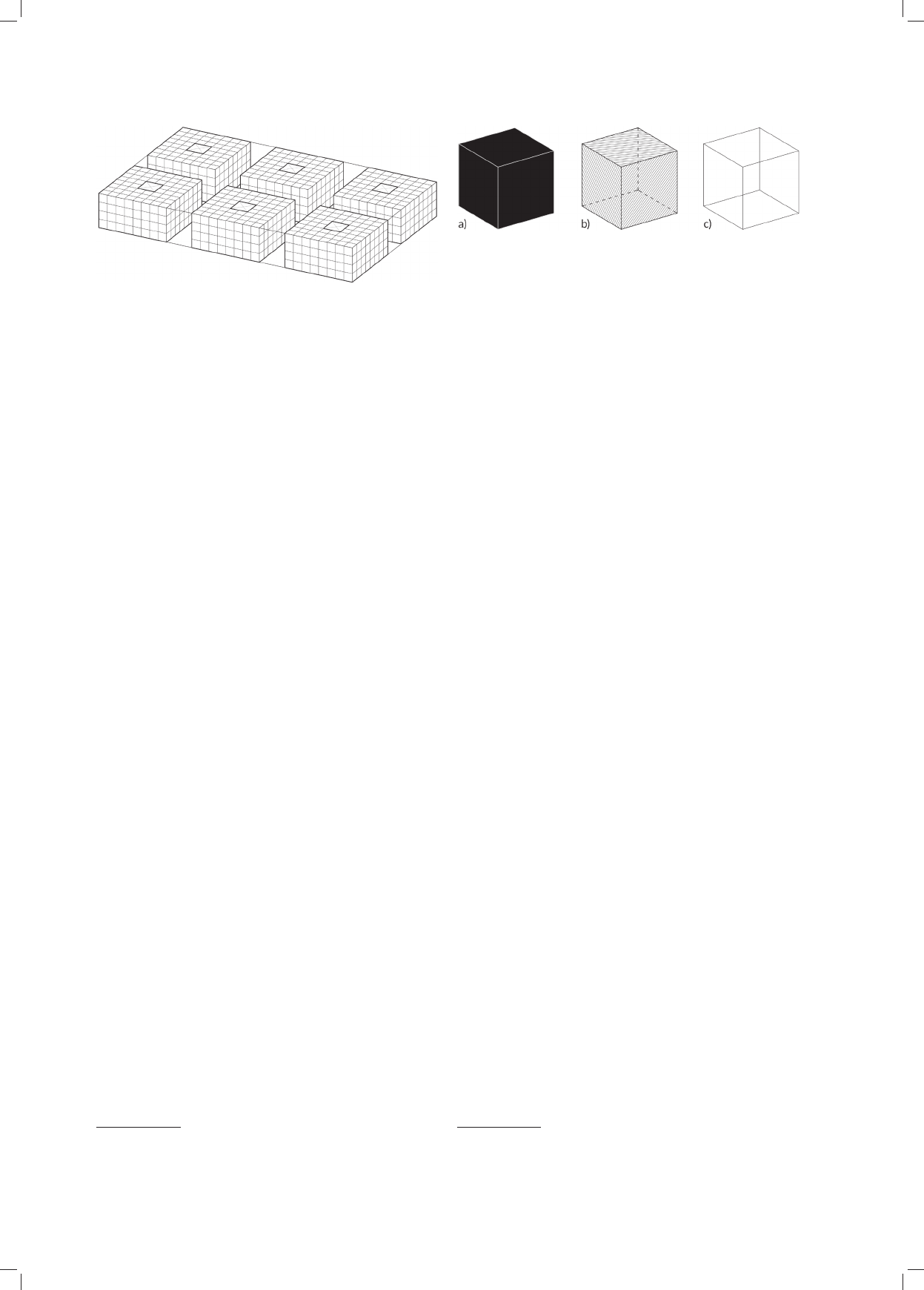
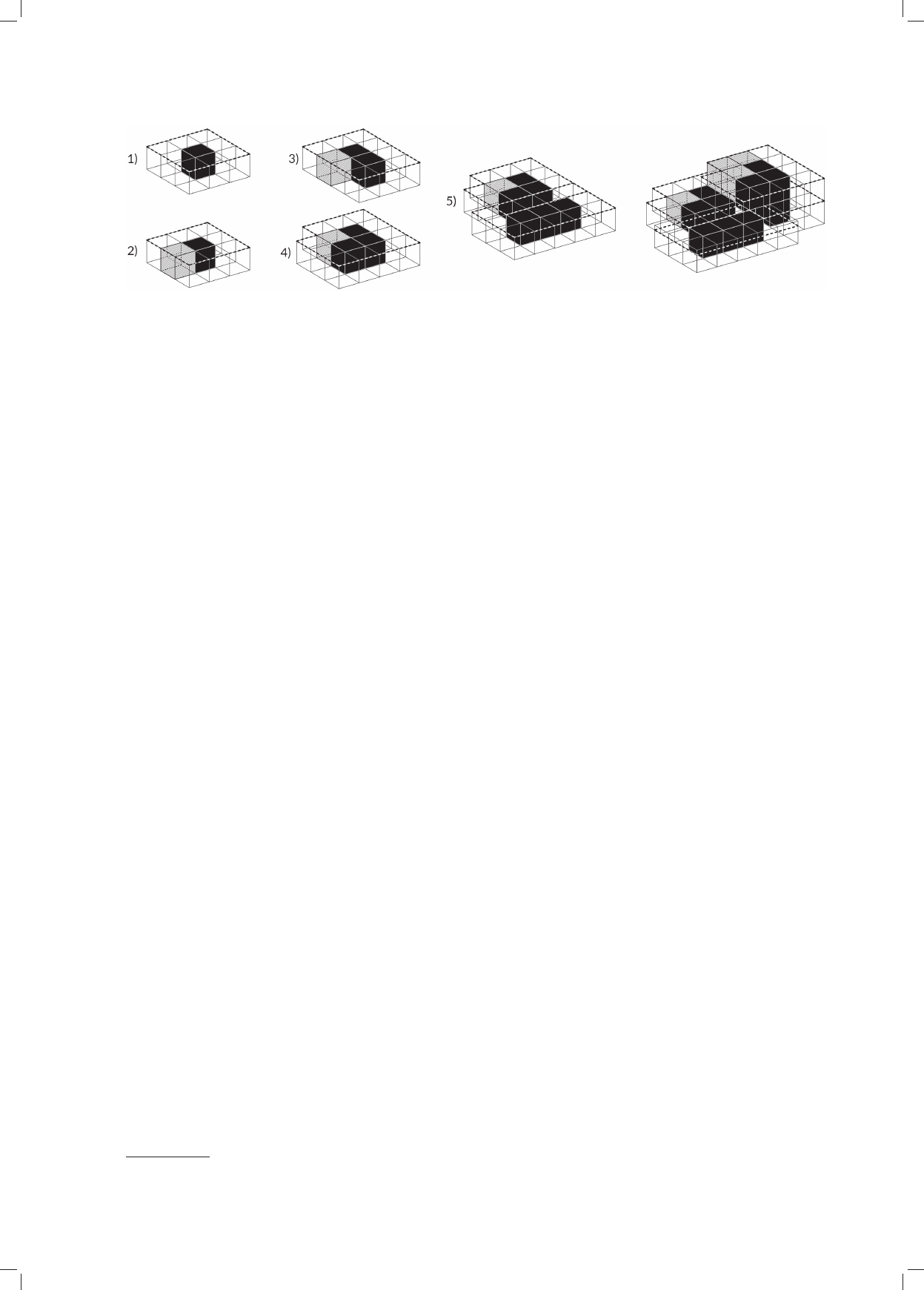
nich struktury stale żywe, jak na przykład Kostki (czte ry
przylegające do siebie komórki żywe), które pozostają
niezmienne w kolejnych cyklach. Oscylatory to struktury
zmienne okresowo, które po określonej liczbie przemian
wracają do swojej pierwotnej konfiguracji. Istnieją także
struktury poruszające się w zorganizowany sposób po
polu gry. Nazywane są Statkami kosmicznymi, a najpopu-
larniejszy z nich to Szybowiec – konfiguracja składa
jąca się z pięciu żywych komórek. Inną grupą struktur są
Działa, a wśród nich Wyrzutnia szybowców – konfi gu
racja zbudowana z 26 żywych komórek, która generu je
nieskończoną wiązkę rytmicznie poruszających się Szy
bowców [3].
Wspomniane konfiguracje, ich kombinacje oraz przy-
padkowe układy komórek przypominają zaawansowane
kolonie żywych organizmów, które rodzą się, replikują, roz-
states of particular cells change. The state of a single cell
after the transformation depends entirely on the state in
which surrounding cells (socalled neighborhood) were
before conversion. During the transformation the states of
all cells are changing simultaneously according to the
rules for a particular cellular automaton [1].
In 1970, the British mathematician John Conway de ve
loped a cellular automaton called the Game of Life. It con-
sists of a set of simple rules that allow generating com-
plex structures and their evolution in subsequent cycles
of transformation. The game board is an infinite plane of
an orthogonal net. All the cells located on it must be in
one of the two permitted states – living or dead cell. The
initial states of all cells are determined by the player by
establishing which of them are alive. Generated structure
is then converted in subsequent cycles. State of a cell in
the next generation is determined by the state of its neigh-
borhood in the previous generation. Neighborhood of
each cell consist of eight adjoining cells – four parallel
and four diagonal [2]. Transformations take place on the
basis of the following principles:
– Birth. A cell that is dead in the generation x, becomes
alive in generation x+1 if exactly three cells from its
neighborhood in generation x were alive.
– Death by overcrowding. A cell which is alive in the
generation x and has one or fewer neighbors in this gen-
eration becomes dead in the generation x+1.
– Death by exposure. A cell which is alive in the gene
ration x and has four or more neighbors in this generation
becomes dead in the generation x+1.
– Survival. A cell that is alive in the generation x sur-
vives alive in the generation x+1 only if in the genera-
tion x it will have two or three neighbors [3].
In addition to the regulation of changes in the chaotic
structures, rules of the Game of life generate multiple con-
figurations characterized by a complex behavior. Among
them there are structures constantly alive such as Blocks
(four adjoining living cells), which remain un changed in
subsequent cycles. Oscillators are periodically vari able
structures that after a certain number of changes come
back to their original configuration. There are also struc-
tures moving in an orderly manner on the game board.
They are
called Spaceships, and the most common is Glider
– configuration consisting of five living cells. Another
group of structures are Guns with Glider Gun among them
– configuration built of 26 living cells which generates an
infinite beam of rhythmically moving Gliders [3].
These configurations, their combinations and random
cell systems resemble an advanced colony of living organ-
isms that are born, replicate, grow, move and die. Sub je
cted to further transformation cycles they evolve spontane-
ously into unpredictable structures often selforga nizing
into specialized systems. However, they are driven by sim
ple rules based on the analysis of the nearest neighborhood.
The spatial game – rules of the Cubic parcellation
The primary objective of the presented project was to
hand over fragments of space of the urban block (parcels)
to the inhabitants, so that within them, they could decide